Answers:
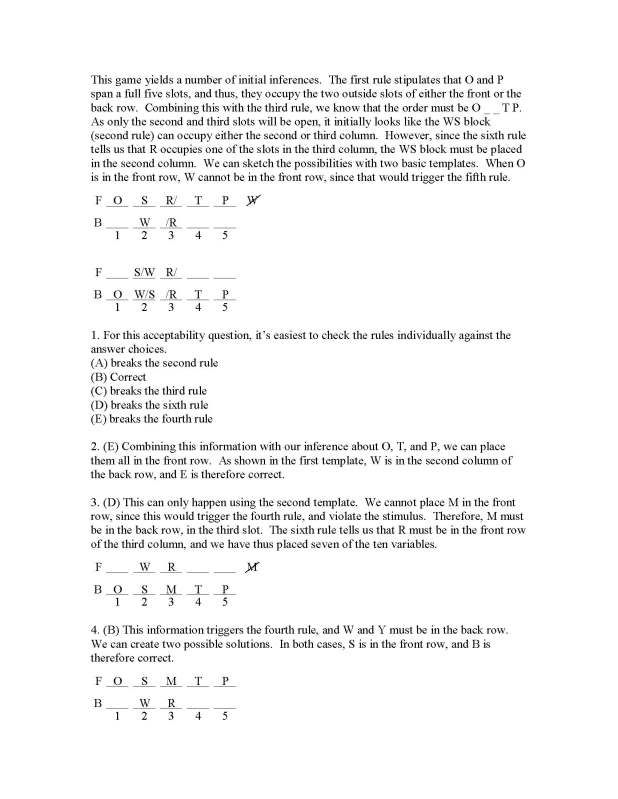
1. B
2. E
3. D
4. B
5. A
6. E
LSAT Forum
15 postsPage 1 of 1
-

- alisasommer
-
Thanks Received: 0
- Forum Guests
- Posts: 2
- Joined: July 09th, 2009
-

- mary.tompkins
-
Thanks Received: 0
- Forum Guests
- Posts: 1
- Joined: June 26th, 2009

- First Responder
Re: Fortnightly Logic Game #10 - The Crayon Game
Explanation and answers:
1. We can quickly eliminate A, because it breaks the 2nd rule - S and W are not in the same column. Next, we can eliminate C, because it breaks rule 3 - T is not directly left of P. The next easy one to eliminate is D, because it breaks the last rule - R is not in the 3rd column. This leaves us with B and E. So, looking at the 2 remaining rules, we can see that the answer must be B, because E breaks the 4th rule - M is in the front row, but so are W and Y.
2. If O is in the first column of the front row, we can diagram this question as:
Front: O _ _ T P, Back: _ _ _ _ _. We know that R must be in the 3rd column, but we do not have enough information to say whether front or back, so A is not the answer. Since we don't know whether R is in the front or back, we cannot say whether M is in the 3rd column in the front or back, so that also eliminates B and C. We have no information about V, so we can eliminate D. This leaves the answer as E, which can be confirmed, since 1) if W were anywhere in the front, O would have to be in the back, 2) S and W must be in the same column, and since R must be in column 3, S and W must be in column 2.
3. Using the same ideas from question 2, we know that if W is in the front row, O must be in the back. From this we can obtain: Front: _ _ _ _ _ (W), Back: O _ _ T P. As stated, R must be in the 3rd column, and S and W are in the same column, which means that we now have: Front: _ W _ _ _, Back: O S _ T P. Looking at rule 4, we can place M in the back row, because if it were in the front, W and Y would need to be in the back, and they cannot be. So now we have: Front: _ W R _ _, Back: O S M T P. This is as far as we can go, because we know nothing about the other 3 crayons, so the answer is D, 7.
4. With M in the front row, we know that w and Y must be in the back row. The only option that we have enough information for is B, that S is in the front row. we know this must be true, because S and W are in the same column, and if W is in the back row, S must therefore be in the front row.
5. If V and Y are in the front row, we know that M cannot be in the front row, as Y would need to be in the back row, so we an eliminate D. We can also eliminate B and C, because if O, V, and Y or T, V, and Y were in the front row, P and T or P and O would also have to be in the front row, and this would mean that S and W could not be in the same column. This means that we know: Front: _ _ _ _ _ (VY), Back: O _ _ T P (M). Since S and W must be in the same column, and R must be in the third column, then: Front: _ _ R _ _ (VY S/W), Back: O _ M T P (S/W). This means we have also eliminated E, because R must be in the front row. Therefore, the only option left is A, S is in the front row.
6. We can eliminate A because knowing that T is in the fourth column of the back row only tells us the positions of O, T, and P. The same can be said of E. B gives us one additional crayon, V, but that still leaves both rows incomplete. We are then left with C and D. The only rule we have pertaining to S is that it must be in the same column as W, which does not give us any information about the others, so C cannot be correct. D must be correct, because if we know that W is in the 2nd column of the front row, then S wil be in the 2nd column of the back row - and if W is in the front row, O must be in the back row, along with T and P. So we have: Front: _ W _ _ _, Back: O S _ T P. We also know that if M is in the front, W and Y would need to be in the back, so M must be in the back. This gives us the complete back row.
1. We can quickly eliminate A, because it breaks the 2nd rule - S and W are not in the same column. Next, we can eliminate C, because it breaks rule 3 - T is not directly left of P. The next easy one to eliminate is D, because it breaks the last rule - R is not in the 3rd column. This leaves us with B and E. So, looking at the 2 remaining rules, we can see that the answer must be B, because E breaks the 4th rule - M is in the front row, but so are W and Y.
2. If O is in the first column of the front row, we can diagram this question as:
Front: O _ _ T P, Back: _ _ _ _ _. We know that R must be in the 3rd column, but we do not have enough information to say whether front or back, so A is not the answer. Since we don't know whether R is in the front or back, we cannot say whether M is in the 3rd column in the front or back, so that also eliminates B and C. We have no information about V, so we can eliminate D. This leaves the answer as E, which can be confirmed, since 1) if W were anywhere in the front, O would have to be in the back, 2) S and W must be in the same column, and since R must be in column 3, S and W must be in column 2.
3. Using the same ideas from question 2, we know that if W is in the front row, O must be in the back. From this we can obtain: Front: _ _ _ _ _ (W), Back: O _ _ T P. As stated, R must be in the 3rd column, and S and W are in the same column, which means that we now have: Front: _ W _ _ _, Back: O S _ T P. Looking at rule 4, we can place M in the back row, because if it were in the front, W and Y would need to be in the back, and they cannot be. So now we have: Front: _ W R _ _, Back: O S M T P. This is as far as we can go, because we know nothing about the other 3 crayons, so the answer is D, 7.
4. With M in the front row, we know that w and Y must be in the back row. The only option that we have enough information for is B, that S is in the front row. we know this must be true, because S and W are in the same column, and if W is in the back row, S must therefore be in the front row.
5. If V and Y are in the front row, we know that M cannot be in the front row, as Y would need to be in the back row, so we an eliminate D. We can also eliminate B and C, because if O, V, and Y or T, V, and Y were in the front row, P and T or P and O would also have to be in the front row, and this would mean that S and W could not be in the same column. This means that we know: Front: _ _ _ _ _ (VY), Back: O _ _ T P (M). Since S and W must be in the same column, and R must be in the third column, then: Front: _ _ R _ _ (VY S/W), Back: O _ M T P (S/W). This means we have also eliminated E, because R must be in the front row. Therefore, the only option left is A, S is in the front row.
6. We can eliminate A because knowing that T is in the fourth column of the back row only tells us the positions of O, T, and P. The same can be said of E. B gives us one additional crayon, V, but that still leaves both rows incomplete. We are then left with C and D. The only rule we have pertaining to S is that it must be in the same column as W, which does not give us any information about the others, so C cannot be correct. D must be correct, because if we know that W is in the 2nd column of the front row, then S wil be in the 2nd column of the back row - and if W is in the front row, O must be in the back row, along with T and P. So we have: Front: _ W _ _ _, Back: O S _ T P. We also know that if M is in the front, W and Y would need to be in the back, so M must be in the back. This gives us the complete back row.
-

- liszerd14
-
Thanks Received: 0
- Forum Guests
- Posts: 1
- Joined: June 22nd, 2009
Re: Fortnightly Logic Game #10 - The Crayon Game
1.B
2. E
3. E
4. B
5. C
6. A
?
2. E
3. E
4. B
5. C
6. A
?
-

- rippinradio
-
Thanks Received: 2
- Forum Guests
- Posts: 24
- Joined: May 02nd, 2009
- This post thanked 1 time.
Re: Fortnightly Logic Game #10 - The Crayon Game

Last edited by rippinradio on Thu Jul 09, 2009 8:05 pm, edited 1 time in total.
-

- igor
-
Thanks Received: 0
-
Vinny Gambini

- Posts: 1
- Joined: July 01st, 2009
Re: Fortnightly Logic Game #10 - The Crayon Game
Because T is directly left of P, and OP are the two ends, we infer that one of the rows contains O1 _ _ T4 P5.
Because R is in the 3rd column, we further infer that S/W are the 2nd column since all other columns have an element assigned.
1.B
A. Breaks SW are the 2nd column
C Breaks OTP are in the same row
D same as A
E Breaks if M front, then W back rule
2.E
The contrapositive of the W front --> O back rule is O front --> W back, which is answer E
3.D
If W2 is front, then O1 is back, along with T4, and P5. S2 is in the same column as W, so S2 is also back.
Since M front would require W back, M must be back, as M3 in the only spot available in the back row. Lastly, R3 must be front since M3 is back.
These add up to 7 known positions.
4.B
M front --> W back --> S front
5.A
V,Y, and either S or W are in front row, and since there are only 5 places OTP must be in the back row.
Because M front --> Y back, M must also be in the back row.
This allows to quickly eliminate choices B,C,D, and E.
6.D
Same reasoning as Q3.
Because R is in the 3rd column, we further infer that S/W are the 2nd column since all other columns have an element assigned.
1.B
A. Breaks SW are the 2nd column
C Breaks OTP are in the same row
D same as A
E Breaks if M front, then W back rule
2.E
The contrapositive of the W front --> O back rule is O front --> W back, which is answer E
3.D
If W2 is front, then O1 is back, along with T4, and P5. S2 is in the same column as W, so S2 is also back.
Since M front would require W back, M must be back, as M3 in the only spot available in the back row. Lastly, R3 must be front since M3 is back.
These add up to 7 known positions.
4.B
M front --> W back --> S front
5.A
V,Y, and either S or W are in front row, and since there are only 5 places OTP must be in the back row.
Because M front --> Y back, M must also be in the back row.
This allows to quickly eliminate choices B,C,D, and E.
6.D
Same reasoning as Q3.
-

- mybiztalk
-
Thanks Received: 0
- Forum Guests
- Posts: 2
- Joined: July 09th, 2009
Re: Fortnightly Logic Game #10 - The Crayon Game
There are 6 Rules to this
1) P _ _ _ O or O _ _ _ P
2) [S] [w]
[W] or[s]
3) [T P]
4) If M
Then W + Y
5) If W
Then O
6) R = 3c
From here we can make a couple of inferences:
1) P _ _ _ O is not possible since we have rule 3 which states T is left of P [T P]
Therefore we can make a giant block O _ _ T P
2) [S]
[W] Column Block must be in Row 2 since 1 4 5 is taken up by the Giant O _ _ T P Block, and we have Rule 6 which states R is in the 3rd Column
3) If M is on front there must be room for W and Y to be on back
4)If O is on front W cannot be on front as well
5) If W or Y is on front then M cannot be on front as well
Question 1)
(A) Front: O,S, R, T, P
Back: W, N, M, Y, V
WRONG violates rule #2.
(B) Front: O, S, R, T, P
Back: N, W, V, Y, M
B is the correct answer it doesnt violate any rules.
(C) Front: Y, W, N, R, V
Back: P, S, M, T, O
WRONG violates Rule #3
(D) Front: O, R, S, T, P
Back: N, V, W, Y, M
WRONG Violates Rule #6
(E) Front: N, W, Y, V, M
Back: O, S, R, T, P
WRONG Violates Rule #6
Question 2. If O is in the first column of the front row, which of the following must be true?
Since this is a Must be true question, 4 answers are not possible and one is possible, so we look at the variable the question is asking of us, which in this case is O so we immediatly know to look at our inferences about O, then find out variable (W in this case) does O affect.
If we look at inference #4 we know that O pushes W to the back row when onfront, and we also know from inference #2 that the S/W Column block must be in row #2, so from here we know that W was pushed to the back row and second column, which is what we see in answer choice E
(A) R is in the third column of the front row.
(B) M is in the third column of the front row.
(C) M is in the third column of the back row.
(D) V is in the fifth column of the back row.
(E) W is in the second column of the back row.
Question 3))))
{If W is in the front row, how many exact rows of crayons can be determined?}
The question stem is asking about the variable W, If we recall there is a two rules regarding W, Rule #2 Rule #5
So we know O has to be on the back (rule 5) and S is in the same column on front of w (rule2)
Next we look through our inferences and look at all of the variables that are in Rule 2 and Rule 5 (O,W, S) and we now know the following:
_ W _ _ _
O S T P
After looking at this we see that there is a constricted space in the back row, if you recall inference #3 we said that if M is on front then we need space for the W and Y, which there isnt any therefore M must take that final spot.
_ W _ _ _
O S M T P
Lastly we know that R must be in column 3 therefore
_ W R _ _
O S M T P
And since we dont know anything about the placement of Y, V, or N (no inferences or rules about them other than knowing v, y must be onfront at this point) we can say the most exact rows we can determine is 7 which is D.
(A) 4
(B) 5
(C) 6
(D) 7
(E) 8
Question 4))))
{If M is in the front row, it must be true that}
Since the question is asking about the variable M we look through the rules and inferences regarding M, which is rule #4 and inference #3
IF M is on front W and Y must be on back and we also know that from Inference #2 regarding the S / W column block, that S is on front of W in column 2, so the answer here is clearly B.
(A) O is in the front row.
(B) S is in the front row.
(C) T is in the back row.
(D) R is in the front row.
(E) R is in the back row.
Question 5))))
If V and Y are in the front row, which of the following could be true?
Here we can look at the rules and inferences for V and Y (rule#4 and inference #5)
We know that M must be on the back because of rule#4 if M was on front then Y is on back which isnt the case so its impossible for M to be on front.
From here we need to check our numerical distribution if V Y and S or W is on front, then we only have 2 spaces left, therefore the Giant O _ _ T P Block is on the back as well, so we can fill in the following. (remember S/w are a column block that goes in column 2 so if ones on back the other is on front, and R must be in column 3)
_ _ R _ _
O _ M T P
So from here we can start crossing out what cant be true
(A) S is in the front row.
(B) O is in the front row. Cant
(C) T is in the front row. Cant
(D) M is in the front row. Cant
(E) R is in the back row. Cant
Were left with A being the only answer which could happen we can put either S or W on front
Question 6))))
Which of the following conditions, if true, would determine the complete order for at least one of the rows?
(A) T is in the fourth column in the back row.
(B) V is in the fourth column of the front row.
(C) S is in the second column in the front row.
(D) W is in the second column in the front row.
(E) P is in the fifth column of the front row.
This question we are looking for the variable which can force an entire row, in order to do this we need to apply all the rules we've had up until this point.
T forces the O _ _ T P block, and the same goes for P... so we can Cross out A and E
V as well forces the O _ _ T P block in the back row, but we still dont know the R or S/W Block that leaves us with C and D.
We know that W forces the O _ _ T P Block in the back row, and since we have no room to plave M on top we have to fill in M into the 3rd column in the back row, therefore D is the right answer.
1) P _ _ _ O or O _ _ _ P
2) [S] [w]
[W] or[s]
3) [T P]
4) If M
Then W + Y
5) If W
Then O
6) R = 3c
From here we can make a couple of inferences:
1) P _ _ _ O is not possible since we have rule 3 which states T is left of P [T P]
Therefore we can make a giant block O _ _ T P
2) [S]
[W] Column Block must be in Row 2 since 1 4 5 is taken up by the Giant O _ _ T P Block, and we have Rule 6 which states R is in the 3rd Column
3) If M is on front there must be room for W and Y to be on back
4)If O is on front W cannot be on front as well
5) If W or Y is on front then M cannot be on front as well
Question 1)
(A) Front: O,S, R, T, P
Back: W, N, M, Y, V
WRONG violates rule #2.
(B) Front: O, S, R, T, P
Back: N, W, V, Y, M
B is the correct answer it doesnt violate any rules.
(C) Front: Y, W, N, R, V
Back: P, S, M, T, O
WRONG violates Rule #3
(D) Front: O, R, S, T, P
Back: N, V, W, Y, M
WRONG Violates Rule #6
(E) Front: N, W, Y, V, M
Back: O, S, R, T, P
WRONG Violates Rule #6
Question 2. If O is in the first column of the front row, which of the following must be true?
Since this is a Must be true question, 4 answers are not possible and one is possible, so we look at the variable the question is asking of us, which in this case is O so we immediatly know to look at our inferences about O, then find out variable (W in this case) does O affect.
If we look at inference #4 we know that O pushes W to the back row when onfront, and we also know from inference #2 that the S/W Column block must be in row #2, so from here we know that W was pushed to the back row and second column, which is what we see in answer choice E
(A) R is in the third column of the front row.
(B) M is in the third column of the front row.
(C) M is in the third column of the back row.
(D) V is in the fifth column of the back row.
(E) W is in the second column of the back row.
Question 3))))
{If W is in the front row, how many exact rows of crayons can be determined?}
The question stem is asking about the variable W, If we recall there is a two rules regarding W, Rule #2 Rule #5
So we know O has to be on the back (rule 5) and S is in the same column on front of w (rule2)
Next we look through our inferences and look at all of the variables that are in Rule 2 and Rule 5 (O,W, S) and we now know the following:
_ W _ _ _
O S T P
After looking at this we see that there is a constricted space in the back row, if you recall inference #3 we said that if M is on front then we need space for the W and Y, which there isnt any therefore M must take that final spot.
_ W _ _ _
O S M T P
Lastly we know that R must be in column 3 therefore
_ W R _ _
O S M T P
And since we dont know anything about the placement of Y, V, or N (no inferences or rules about them other than knowing v, y must be onfront at this point) we can say the most exact rows we can determine is 7 which is D.
(A) 4
(B) 5
(C) 6
(D) 7
(E) 8
Question 4))))
{If M is in the front row, it must be true that}
Since the question is asking about the variable M we look through the rules and inferences regarding M, which is rule #4 and inference #3
IF M is on front W and Y must be on back and we also know that from Inference #2 regarding the S / W column block, that S is on front of W in column 2, so the answer here is clearly B.
(A) O is in the front row.
(B) S is in the front row.
(C) T is in the back row.
(D) R is in the front row.
(E) R is in the back row.
Question 5))))
If V and Y are in the front row, which of the following could be true?
Here we can look at the rules and inferences for V and Y (rule#4 and inference #5)
We know that M must be on the back because of rule#4 if M was on front then Y is on back which isnt the case so its impossible for M to be on front.
From here we need to check our numerical distribution if V Y and S or W is on front, then we only have 2 spaces left, therefore the Giant O _ _ T P Block is on the back as well, so we can fill in the following. (remember S/w are a column block that goes in column 2 so if ones on back the other is on front, and R must be in column 3)
_ _ R _ _
O _ M T P
So from here we can start crossing out what cant be true
(A) S is in the front row.
(B) O is in the front row. Cant
(C) T is in the front row. Cant
(D) M is in the front row. Cant
(E) R is in the back row. Cant
Were left with A being the only answer which could happen we can put either S or W on front
Question 6))))
Which of the following conditions, if true, would determine the complete order for at least one of the rows?
(A) T is in the fourth column in the back row.
(B) V is in the fourth column of the front row.
(C) S is in the second column in the front row.
(D) W is in the second column in the front row.
(E) P is in the fifth column of the front row.
This question we are looking for the variable which can force an entire row, in order to do this we need to apply all the rules we've had up until this point.
T forces the O _ _ T P block, and the same goes for P... so we can Cross out A and E
V as well forces the O _ _ T P block in the back row, but we still dont know the R or S/W Block that leaves us with C and D.
We know that W forces the O _ _ T P Block in the back row, and since we have no room to plave M on top we have to fill in M into the 3rd column in the back row, therefore D is the right answer.
-

- soumyaak
-
Thanks Received: 0
- Forum Guests
- Posts: 1
- Joined: July 09th, 2009
Re: Fortnightly Logic Game #10 - The Crayon Game
My answers to the crayon challenge are
1. B
2. E
3. B
4. B
5. A
6. D
Thanks!
1. B
2. E
3. B
4. B
5. A
6. D
Thanks!
-

- rippinradio
-
Thanks Received: 2
- Forum Guests
- Posts: 24
- Joined: May 02nd, 2009
Re: Fortnightly Logic Game #10 - The Crayon Game
7. If R and T are both in the front row, how many unique pairings of crayons to positions are there?
(A) 6
(B) 7
(C) 8
(D) 9
(E) 10
(A) 6
(B) 7
(C) 8
(D) 9
(E) 10
-

- noah
-
Thanks Received: 1192
-
Atticus Finch

- Posts: 1541
- Joined: February 11th, 2009
Re: Fortnightly Logic Game #10 - The Crayon Game
rippinradio Wrote:7. If R and T are both in the front row, how many unique pairings of crayons to positions are there?
(A) 6
(B) 7
(C) 8
(D) 9
(E) 10
Bravo Rippinradio! I'm going to solve this question pronto!
-

- timmacgougan
-
Thanks Received: 0
-
Vinny Gambini

- Posts: 2
- Joined: May 19th, 2009
Re: Fortnightly Logic Game #10 - The Crayon Game
Setup: The best way to set this up is with 2 rows of 5 lines, a line for each slot. We can place R beneath the 3rd column. We know that O and P are on the outsides, and that T is to the left of P, so O must be 1st, T 4th, and P in the 5th slot. Furthermore, we know that O, T and P will all be in the same row. Since we know that W and S are in the same column, they have to both be in column 2. Lastly, written or unwritten, it’s good to know that leaves us with M and Y who have conditional constraints; N and V are floaters.
_ _ _ _ _
_ _ _ _ _
O W/S R T P
1. This is an orientation question, the best way to solve it as this class has taught, is to go down the list of constraints finding the answer that betrays each one. We are left with B.
2. This is a "which must be true" question so the goal should be to find the correct answer, not to weed out the incorrect answers, because the answer will be the only choice that has certainty. The first thing to do is to find the answers with P and W, because they have known direct relationships with O. In this case, there are no answers with P, and the answer with W happens to be correct: convenient. It is correct because we know that W is in the second column from our setup, and it can’t be in the front row because then O would have to be in the back, which we know it isn’t. E, No need to test other answers.
3. 1: W is given to us in the front row, and we know it’s in the second column. 2: S is known to be in the second column, so it must be in the back row. 3: O must be in the back row because W is in front, it goes in the 1st slot. 4&5: T and P must be in the 4th and 5th columns of the back row in order to be in the same row as O. 6: Now the best thing to do is notice that there’s only one slot in the back, and we can see that M can’t be on top because W can’t be in the back, so M must take that spot, 3rd column. 7: Now R must take the remaining 3rd column spot in the front.
Y is the only letter left with a constraint, and its "If" statement isn’t activated so we can ignore that. So now N, V and Y are floaters and can go anywhere. 7 definite spots: answer D.
4. With M in the front, we know W (and Y) is in the back, and therefore S must be in the front. This gives only two definite positions, S&W in the second column. It’s not much to go by but the answer is there. Answer: B.
5. The best starting point here is that we know that the O_ _TP combo must be in the back row because there isn’t room for it up front along with V, Y, and (W or S). Next, we know W must be in back because Y is in front, and therefore S is in front. Then we place M in the back row because Y is in front, it must go in the 3rd column, so that bumps R up front in the 3rd column. Taking a look at the answers at this point reveals that all but A have been eliminated. Answer A.
6. Problem 3 gives the answer. W was in front, in the second column, and it provided us the entire bottom row. Answer D.
7. Additional Question. Which constraint, if applied, yields the most possible arrangements of crayons?
a. N and V are in consecutive columns in different rows
b. N and V are in consecutive columns in the same row
c. N and V are in the same row, and separated by exactly 1 column
d. N and V are in the same row, and separated by exactly 2 columns
e. N and V are in the same row, and separated by exactly 3 columns
_ _ _ _ _
_ _ _ _ _
O W/S R T P
1. This is an orientation question, the best way to solve it as this class has taught, is to go down the list of constraints finding the answer that betrays each one. We are left with B.
2. This is a "which must be true" question so the goal should be to find the correct answer, not to weed out the incorrect answers, because the answer will be the only choice that has certainty. The first thing to do is to find the answers with P and W, because they have known direct relationships with O. In this case, there are no answers with P, and the answer with W happens to be correct: convenient. It is correct because we know that W is in the second column from our setup, and it can’t be in the front row because then O would have to be in the back, which we know it isn’t. E, No need to test other answers.
3. 1: W is given to us in the front row, and we know it’s in the second column. 2: S is known to be in the second column, so it must be in the back row. 3: O must be in the back row because W is in front, it goes in the 1st slot. 4&5: T and P must be in the 4th and 5th columns of the back row in order to be in the same row as O. 6: Now the best thing to do is notice that there’s only one slot in the back, and we can see that M can’t be on top because W can’t be in the back, so M must take that spot, 3rd column. 7: Now R must take the remaining 3rd column spot in the front.
Y is the only letter left with a constraint, and its "If" statement isn’t activated so we can ignore that. So now N, V and Y are floaters and can go anywhere. 7 definite spots: answer D.
4. With M in the front, we know W (and Y) is in the back, and therefore S must be in the front. This gives only two definite positions, S&W in the second column. It’s not much to go by but the answer is there. Answer: B.
5. The best starting point here is that we know that the O_ _TP combo must be in the back row because there isn’t room for it up front along with V, Y, and (W or S). Next, we know W must be in back because Y is in front, and therefore S is in front. Then we place M in the back row because Y is in front, it must go in the 3rd column, so that bumps R up front in the 3rd column. Taking a look at the answers at this point reveals that all but A have been eliminated. Answer A.
6. Problem 3 gives the answer. W was in front, in the second column, and it provided us the entire bottom row. Answer D.
7. Additional Question. Which constraint, if applied, yields the most possible arrangements of crayons?
a. N and V are in consecutive columns in different rows
b. N and V are in consecutive columns in the same row
c. N and V are in the same row, and separated by exactly 1 column
d. N and V are in the same row, and separated by exactly 2 columns
e. N and V are in the same row, and separated by exactly 3 columns
-

- rippinradio
-
Thanks Received: 2
- Forum Guests
- Posts: 24
- Joined: May 02nd, 2009
Re: Fortnightly Logic Game #10 - The Crayon Game
That's a great question! I got B for number 7.
-

- jdillier
-
Thanks Received: 0
-
Vinny Gambini

- Posts: 5
- Joined: February 15th, 2009

- First Responder
Re: Fortnightly Logic Game #10 - The Crayon Game
A crayon manufacturer is designing a box with ten colors of crayons _ M, N, O, P, R, S, T, V, W, and Y"”which will be arranged in two rows"”front and back"”and five columns, labeled 1-5 from left to right.
This asks to assign crayons to rows and columns. The diagram will look like this.
Back Row ___ ___ ___ ___ ___ ___
Front Row___ ___ ___ ___ ___ ___
1 2 3 4 5 6
The following conditions apply:
P is in the same row as O, and there are exactly three slots between them.
Notate by P __ ___ __ O or O __ __ __ P
S is in the same column as W.
Notate by putting S over W like: S or W
W S
T is directly to the left of P.
This constraint says (TP) So, we now that O __ __ T P. This is a big chunk. We can also forget the possibility of P __ __ __ O because there would be no where to place T
If M is in the front row, W and Y will be in the back row.
M (frt) "”> W & Y (bck)
If W is in the front row, O will be in the back row.
W (frt) "”> O (bck)
R is in the third column.
R=3 Notate by putting R about column 3 to make it "˜float’
1st Diagram:
M, N, O, P, R, S, T, V, W, and Y
(R)
Back Row ___ ___ ___ ___ ___ S or W O ___ ___ T P
Front Row___ ___ ___ ___ ___ W S
1 2 3 4 5
M (frt) "”> W & Y (bck)
W (frt) "”-> O (bck)
1. Which of the following could be a possible arrangement of crayons, from left to right?
This problem is best answered by taking each constraint and applying it to each answer choice and then eliminating wrong choices.
(A) Front: O,S, R, T, P eliminate because S and W must be in the same column
     Back: W, N, M, Y, V
(B) Front: O, S, R, T, P correct answer!
     Back: N, W, V, Y, M
(C) Front: Y, W, N, R, V eliminate because T must be directly left of P
     Back: P, S, M, T, O
(D) Front: O, R, S, T, P eliminate because R is not in the 3rd column
     Back: N, V, W, Y, M
(E) Front: N, W, Y, V, M eliminate because if M is in the front Y and V must be in the back
     Back: O, S, R, T, P
2. If O is in the first column of the front row, which of the following must be true?
(A) R is in the third column of the front row.
(B) M is in the third column of the front row.
(C) M is in the third column of the back row.
(D) V is in the fifth column of the back row.
(E) W is in the second column of the back row. Correct!
This requires a new diagram:
First place O in the first column in the front row. We now can see the rest of that chunk must fit in the front row. T and P go in 4 and 5 in the front row. This still does not give enough information to answer the question though.
Back Row ___ ___ ___ ___ ___ S or W O ___ ___ T P
Front Row O __ ___ T P W S
1 2 3 4 5
Now we still know that S and W must have a column all to themselves so this must be column 2 but we are not sure which row each must go in. BUT WAIT, if W were to be in the front row then O would be forced to the back which can’t be true. Thus we know W will be in the back and S in the front of column 2!
Final Diagram
(R)
Back Row ___ W ___ ___ ___ S or W O ___ ___ T P
Front Row O S ___ T P W S
1 2 3 4 5
M (frt) "”> W & Y (bck)
W (frt) "”-> O (bck)
3. If W is in the front row, how many exact positions of crayons can be determined?
(A) 4
(B) 5
(C) 6
(D) 7 correct!
(E) 8
For this "IF" question again we will need a new diagram for the condition.
(R)
Back Row ___ ___ ___ ___ ___
Front Row___ ___ ___ ___ ___ W
1 2 3 4 5
We know from the constraints that is W is in the front then O must be in the back. Which will also put T and P in the back as well.
(R)
Back Row O ___ ___ T P
Front Row___ ___ ___ ___ ___ W
1 2 3 4 5
Because of the S/W constraint S must also be in the back row because it will be in the same column as W. There are two open spots, 2 and 3 and at least one spot in column 3 will be for R so the S and W column combination will be in column 2.
(R)
Back Row O S ___ T P
Front Row___ W ___ ___ ___
1 2 3 4 5
We also know that if M were to be in the front row W and Y would be in the back. Not possible here so M will go in the final spot in column 3 in the back row and R will be in the front row of column 3. These are the only inference to be make so the answer is B) 7.
Back Row O S M T P
Front Row___ W R ___ ___
1 2 3 4 5
4. If M is in the front row, it must be true that
(A) O is in the front row.
(B) S is in the front row. Correct!
(C) T is in the back row.
(D) R is in the front row.
(E) R is in the back row.
For this question we will build a new diagram with the "IF" statement included. Place the M out to the side of row one since we are still unsure which column it will fit in.
Back Row ___ ___ ___ ___ ___
Front Row___ ___ ___ ___ ___ M
1 2 3 4 5
We know from the constraints that if M is in the front then W and Y will be in the back. If W is in the back then S must be in the front because they must be in the same column. B is the correct answer!
Back Row ___ ___ ___ ___ ___ Y, W
Front Row___ ___ ___ ___ ___ M, S
1 2 3 4 5
5. If V and Y are in the front row, which of the following could be true?
(A) S is in the front row.
(B) O is in the front row.
(C) T is in the front row.
(D) M is in the front row.
(E) R is in the back row.
We are looking to eliminate answers that must be false. Again, we must build another diagram with V and Y in the front row.
Back Row ___ ___ ___ ___ ___
Front Row___ ___ ___ ___ ___ V, Y
1 2 3 4 5
If Y is in front then we know for sure M is in the back since putting it in front would violate the M (frt) "”> W & Y (bck) rule.
Back Row ___ ___ ___ ___ ___ M
Front Row___ ___ ___ ___ ___ V, Y
1 2 3 4 5
We can eliminate D.
There are no other inferences here so we are forced to use trial and error.
Starting with A it doesn’t seem to violate any rules so it could be true and the answer is A! Just to be sure here is why B, C and E are wrong.
B_ O is in front and C_ T is in front
Back Row ___ ___ ___ ___ ___ M
Front Row O ___ ___ T P V, Y
1 2 3 4 5
There is now no room for the S/W column so O, T, and P must be in the back row.
E_ R is in the back.
Now we finally know O,T and P are in the back. Remembering the S/W column there would be no room for O, T, P, M, R and S/W.
Back Row O ___ ___ T P M,
Front Row ___ ___ ___ ___ ___ V, Y
1 2 3 4 5
6. Which of the following conditions, if true, would determine the complete order for at least one of the rows?
(A) T is in the fourth column in the back row.
(B) V is in the fourth column of the front row.
(C) S is in the second column in the front row.
(D) W is in the second column in the front row. Correct!
(E) P is in the fifth column of the front row.
The best way to attempt this is with a time killing trial and error approach. But the bright side is by this point the constraints and inferences are familiar.
(R)
A) Back Row O W ___ T P
Front Row __ S ___ ___ ___
1 2 3 4 5
This can’t be continued any farther because either M or Y will go in the back of the 3rd column.
(R)
B) Back Row ___ ___ ___ ___ ___
Front Row___ ___ ___ V ___
1 2 3 4 5
V does not lead us anywhere.
(R)
C) Back Row ___ W ___ ___ ___
Front Row___ S ___ ___ ___
1 2 3 4 5
This does not fill a row either.
(R)
D) Back Row O S M T P
Front Row___ W ___ ___ ___
1 2 3 4 5
With W in the 2nd column in the front we know that S will go in the back of the 2nd column. Now if W is in the front O along with it’s chunk T and P will also go in the back. M will also be forced in the back too in the final spot because if it were in the front it would violate the M (frt) "”> W & Y (bck) constraint. D is the answer.
(R)
E) Back Row ___ ___ ___ ___ ___
Front Row O ___ ___ T P
1 2 3 4 5
This does not fill in a row either.
This asks to assign crayons to rows and columns. The diagram will look like this.
Back Row ___ ___ ___ ___ ___ ___
Front Row___ ___ ___ ___ ___ ___
1 2 3 4 5 6
The following conditions apply:
P is in the same row as O, and there are exactly three slots between them.
Notate by P __ ___ __ O or O __ __ __ P
S is in the same column as W.
Notate by putting S over W like: S or W
W S
T is directly to the left of P.
This constraint says (TP) So, we now that O __ __ T P. This is a big chunk. We can also forget the possibility of P __ __ __ O because there would be no where to place T
If M is in the front row, W and Y will be in the back row.
M (frt) "”> W & Y (bck)
If W is in the front row, O will be in the back row.
W (frt) "”> O (bck)
R is in the third column.
R=3 Notate by putting R about column 3 to make it "˜float’
1st Diagram:
M, N, O, P, R, S, T, V, W, and Y
(R)
Back Row ___ ___ ___ ___ ___ S or W O ___ ___ T P
Front Row___ ___ ___ ___ ___ W S
1 2 3 4 5
M (frt) "”> W & Y (bck)
W (frt) "”-> O (bck)
1. Which of the following could be a possible arrangement of crayons, from left to right?
This problem is best answered by taking each constraint and applying it to each answer choice and then eliminating wrong choices.
(A) Front: O,S, R, T, P eliminate because S and W must be in the same column
     Back: W, N, M, Y, V
(B) Front: O, S, R, T, P correct answer!
     Back: N, W, V, Y, M
(C) Front: Y, W, N, R, V eliminate because T must be directly left of P
     Back: P, S, M, T, O
(D) Front: O, R, S, T, P eliminate because R is not in the 3rd column
     Back: N, V, W, Y, M
(E) Front: N, W, Y, V, M eliminate because if M is in the front Y and V must be in the back
     Back: O, S, R, T, P
2. If O is in the first column of the front row, which of the following must be true?
(A) R is in the third column of the front row.
(B) M is in the third column of the front row.
(C) M is in the third column of the back row.
(D) V is in the fifth column of the back row.
(E) W is in the second column of the back row. Correct!
This requires a new diagram:
First place O in the first column in the front row. We now can see the rest of that chunk must fit in the front row. T and P go in 4 and 5 in the front row. This still does not give enough information to answer the question though.
Back Row ___ ___ ___ ___ ___ S or W O ___ ___ T P
Front Row O __ ___ T P W S
1 2 3 4 5
Now we still know that S and W must have a column all to themselves so this must be column 2 but we are not sure which row each must go in. BUT WAIT, if W were to be in the front row then O would be forced to the back which can’t be true. Thus we know W will be in the back and S in the front of column 2!
Final Diagram
(R)
Back Row ___ W ___ ___ ___ S or W O ___ ___ T P
Front Row O S ___ T P W S
1 2 3 4 5
M (frt) "”> W & Y (bck)
W (frt) "”-> O (bck)
3. If W is in the front row, how many exact positions of crayons can be determined?
(A) 4
(B) 5
(C) 6
(D) 7 correct!
(E) 8
For this "IF" question again we will need a new diagram for the condition.
(R)
Back Row ___ ___ ___ ___ ___
Front Row___ ___ ___ ___ ___ W
1 2 3 4 5
We know from the constraints that is W is in the front then O must be in the back. Which will also put T and P in the back as well.
(R)
Back Row O ___ ___ T P
Front Row___ ___ ___ ___ ___ W
1 2 3 4 5
Because of the S/W constraint S must also be in the back row because it will be in the same column as W. There are two open spots, 2 and 3 and at least one spot in column 3 will be for R so the S and W column combination will be in column 2.
(R)
Back Row O S ___ T P
Front Row___ W ___ ___ ___
1 2 3 4 5
We also know that if M were to be in the front row W and Y would be in the back. Not possible here so M will go in the final spot in column 3 in the back row and R will be in the front row of column 3. These are the only inference to be make so the answer is B) 7.
Back Row O S M T P
Front Row___ W R ___ ___
1 2 3 4 5
4. If M is in the front row, it must be true that
(A) O is in the front row.
(B) S is in the front row. Correct!
(C) T is in the back row.
(D) R is in the front row.
(E) R is in the back row.
For this question we will build a new diagram with the "IF" statement included. Place the M out to the side of row one since we are still unsure which column it will fit in.
Back Row ___ ___ ___ ___ ___
Front Row___ ___ ___ ___ ___ M
1 2 3 4 5
We know from the constraints that if M is in the front then W and Y will be in the back. If W is in the back then S must be in the front because they must be in the same column. B is the correct answer!
Back Row ___ ___ ___ ___ ___ Y, W
Front Row___ ___ ___ ___ ___ M, S
1 2 3 4 5
5. If V and Y are in the front row, which of the following could be true?
(A) S is in the front row.
(B) O is in the front row.
(C) T is in the front row.
(D) M is in the front row.
(E) R is in the back row.
We are looking to eliminate answers that must be false. Again, we must build another diagram with V and Y in the front row.
Back Row ___ ___ ___ ___ ___
Front Row___ ___ ___ ___ ___ V, Y
1 2 3 4 5
If Y is in front then we know for sure M is in the back since putting it in front would violate the M (frt) "”> W & Y (bck) rule.
Back Row ___ ___ ___ ___ ___ M
Front Row___ ___ ___ ___ ___ V, Y
1 2 3 4 5
We can eliminate D.
There are no other inferences here so we are forced to use trial and error.
Starting with A it doesn’t seem to violate any rules so it could be true and the answer is A! Just to be sure here is why B, C and E are wrong.
B_ O is in front and C_ T is in front
Back Row ___ ___ ___ ___ ___ M
Front Row O ___ ___ T P V, Y
1 2 3 4 5
There is now no room for the S/W column so O, T, and P must be in the back row.
E_ R is in the back.
Now we finally know O,T and P are in the back. Remembering the S/W column there would be no room for O, T, P, M, R and S/W.
Back Row O ___ ___ T P M,
Front Row ___ ___ ___ ___ ___ V, Y
1 2 3 4 5
6. Which of the following conditions, if true, would determine the complete order for at least one of the rows?
(A) T is in the fourth column in the back row.
(B) V is in the fourth column of the front row.
(C) S is in the second column in the front row.
(D) W is in the second column in the front row. Correct!
(E) P is in the fifth column of the front row.
The best way to attempt this is with a time killing trial and error approach. But the bright side is by this point the constraints and inferences are familiar.
(R)
A) Back Row O W ___ T P
Front Row __ S ___ ___ ___
1 2 3 4 5
This can’t be continued any farther because either M or Y will go in the back of the 3rd column.
(R)
B) Back Row ___ ___ ___ ___ ___
Front Row___ ___ ___ V ___
1 2 3 4 5
V does not lead us anywhere.
(R)
C) Back Row ___ W ___ ___ ___
Front Row___ S ___ ___ ___
1 2 3 4 5
This does not fill a row either.
(R)
D) Back Row O S M T P
Front Row___ W ___ ___ ___
1 2 3 4 5
With W in the 2nd column in the front we know that S will go in the back of the 2nd column. Now if W is in the front O along with it’s chunk T and P will also go in the back. M will also be forced in the back too in the final spot because if it were in the front it would violate the M (frt) "”> W & Y (bck) constraint. D is the answer.
(R)
E) Back Row ___ ___ ___ ___ ___
Front Row O ___ ___ T P
1 2 3 4 5
This does not fill in a row either.
-

- summerskyz21
-
Thanks Received: 0
- Forum Guests
- Posts: 1
- Joined: July 07th, 2009
Here's the answers I got for the Crayon Logic Game..
1. B
2. E
3. B
4. B
5. A
6. D
2. E
3. B
4. B
5. A
6. D
-

- noah
-
Thanks Received: 1192
-
Atticus Finch

- Posts: 1541
- Joined: February 11th, 2009
Re: Here's the answers I got for the Crayon Logic Game..
Hey There, You're pretty close...Just #3 is incorrect. Want to try again? You can look at some explanations here: fortnightly-logic-game-10-the-crayon-game-t202.html
Can you figure out what your mistake in thinking was?
Good luck!
- Noah
Can you figure out what your mistake in thinking was?
Good luck!
- Noah
-

- martin.x.mitchell
-
Thanks Received: 0
- Forum Guests
- Posts: 1
- Joined: July 15th, 2009
Re: Fortnightly Logic Game #10 - The Crayon Game
Before I went through the questions I did a quick assessment to see how many were Global problems, which would tell me that I had to spend more time on the set-up. Since most of them are local the set-up was quick and I knew that there wouldn't be too many inferences.
1) Ordering questions. Just plug in the rules. B is answer.
I first looked at the OP rule. O is first and P is last and T is Fourth (per rule 3)
Answer C is out because it's not O-T-P. it's P first and O last.
Next I looked at the SW block.
Answer A is out because S and W are not the same column.
Then I went to rule 6 since it was easier to manage then the conditionals.
Answer D is out because R is not in the 3rd column.
I did rule 5 next, the easier of the conditionals
Answer E is the only one not eliminated that has W in front, but it also has O in the back so it may be right, so I went to the next rule.
Rule 4 which is two conditionals in one.
Answer E is out because M is in the front but so is W and Y and the rule has both of them in the back
The only answer that hasn't been eliminated is B
2) Local, Must be True and conditional. E is answer.
I plugged O in the front row as stated in the question stem, T in the 4th column and P in the last column both in the front row.
So there are two slots left. But R is in the 3rd slot and SW have to be in the same column so SW have to go in slot 2 since R is in slot 3. Then I got to rule #5 where if W is in the front row then O is in the back row, but O is in the front row, not in the back row which is the contrapositive [if not O in back then not W in front]. W is not in front meaning that it has to be in the back, and S has to be in the front. E has W in the front row.
3) Local, Can Be true, Conditional. D is the answer.
Same as question 2 where I make a local diagram with W. I then use the main diagram that has S/W going in slot 2 and since the question stem has W in the front row it goes in slot 2 in the front, with S in slot 2 in the back. Looking at rule 5: If W is in the front then the OTP superblock is in the back. Then I look at rule 4 that says that if M is in the front then W is in the back (and Y also, but Y is a floater so it has no power--and I ignore it since I made two separate rules for rule 4). But since the stem states that W must be in the front we go to the contrapostitive of rule 4 that says if not W in the back then not M in the front. Since W is not in the back M cannot be in the front and the only other place for it is in the back row. There is only one slot left in the back row open and that is slot 3--we dump M in here and since R must be in the 3rd slot the only place for R to go is in the front row, 3rd slot. So now we have determined slots 1,2,3,4,5-back and slots 2,3-front. There are 3 open slots in the front and 3 variables but they do not have any rules allotted to them so they cannot be placed in any set position. We have determined 7 slots as stated in answer D.
4) Local, conditional, must be true. B is the answer.
Make local diagram with M in front row, written off to the side.
Plug in rule 4 that says that M is in front and W and Y are in back. From the main diagram we know that W can only go in 2 and S has to be in the same column.
I glanced the answers after I had the diagram and saw that S must be in the front row, because if W is in the back only S can be in the same column.
5) Local, conditional, could be true. A is the answer.
V and Y in the front row means that M could not be in the front row, as this would violate rule 4. Answer D is eliminated
So with V and Y in the front and M in the back I do a local diagram.
I did two hypotheticals with the OTP superblock (one with the block in the front row and one with it in the back row)
Since slots 1,4, and 5 are taken by OTP respectively there are only two places where V and Y can go, in slots 2 and 3, but then there is no place for either S or W to go and one of them has to be in 2 (front the main diagram) so the OTP superblock cannot go in front, it must go in back. Answers B and C are eliminated.
Only answers E and A remain so I make two hypotheticals. I already know that the OTP block must be in the back.
With OTP in the back, remember that M must also go in the back so there has to be an extra slot for it.
If R is in the back then that would mean the 1,3,4, and 5 slots are taken and M must go in 2, but either S or W must go in two so this cannot work. Eliminate E.
6) This is the drainer, but I'm ready. I know that most likely I will have to make 5 hypotheticals for this one, so I budgeted my time accordingly. D is the answer
T is the fourth column in the back row. That would mean that the OTP superblock is in the back row as well. Since R has to be in the 3rd row, either S/W must be in the second slot. R could be in the front row or the back row and either S/W can be in the slot 2 so we can't get a complete order. Eliminate A.
V is in the forth column of the front row. Since V is in the fourth slot in the front row that means that T is in the back row in the fourth slot (per rule 3) so that would mean that the OTP superblock is in the back row. R must be in the third row and S/W in the second row but we don't exactly know where these three variables go. Since we have not determined where three of the variables go we don't have a complete row settled . Eliminate B.
S is in the second column of the front row. When S is in the second column of the front row that means that W is in the second slot in the back row. But the OTP superblock can either go in the front or the back. This alone does not delineate an entire row. Eliminate C
W is in the second column of the first row. When W is in the second column of the first row then S is in the second column of the back row.
Then we look at rule 5 that says that O must be in the back row when W is in the front. Plug in the OTP superblock in the back row and slots 1,2,4, and 5 are determined. Only 3 remains. This is the trick--Since W is in front then rule 4 is violated if M is also in the front, M cannot go in the front; then W would have to go in the back and it does not. So that means that M, not in the front, must be in the back and the only place where it could possibly go is in the 3rd slot. So we have a completed row of OWMTP. D is the correct answer
Cheers,
Marty Mitchell
1) Ordering questions. Just plug in the rules. B is answer.
I first looked at the OP rule. O is first and P is last and T is Fourth (per rule 3)
Answer C is out because it's not O-T-P. it's P first and O last.
Next I looked at the SW block.
Answer A is out because S and W are not the same column.
Then I went to rule 6 since it was easier to manage then the conditionals.
Answer D is out because R is not in the 3rd column.
I did rule 5 next, the easier of the conditionals
Answer E is the only one not eliminated that has W in front, but it also has O in the back so it may be right, so I went to the next rule.
Rule 4 which is two conditionals in one.
Answer E is out because M is in the front but so is W and Y and the rule has both of them in the back
The only answer that hasn't been eliminated is B
2) Local, Must be True and conditional. E is answer.
I plugged O in the front row as stated in the question stem, T in the 4th column and P in the last column both in the front row.
So there are two slots left. But R is in the 3rd slot and SW have to be in the same column so SW have to go in slot 2 since R is in slot 3. Then I got to rule #5 where if W is in the front row then O is in the back row, but O is in the front row, not in the back row which is the contrapositive [if not O in back then not W in front]. W is not in front meaning that it has to be in the back, and S has to be in the front. E has W in the front row.
3) Local, Can Be true, Conditional. D is the answer.
Same as question 2 where I make a local diagram with W. I then use the main diagram that has S/W going in slot 2 and since the question stem has W in the front row it goes in slot 2 in the front, with S in slot 2 in the back. Looking at rule 5: If W is in the front then the OTP superblock is in the back. Then I look at rule 4 that says that if M is in the front then W is in the back (and Y also, but Y is a floater so it has no power--and I ignore it since I made two separate rules for rule 4). But since the stem states that W must be in the front we go to the contrapostitive of rule 4 that says if not W in the back then not M in the front. Since W is not in the back M cannot be in the front and the only other place for it is in the back row. There is only one slot left in the back row open and that is slot 3--we dump M in here and since R must be in the 3rd slot the only place for R to go is in the front row, 3rd slot. So now we have determined slots 1,2,3,4,5-back and slots 2,3-front. There are 3 open slots in the front and 3 variables but they do not have any rules allotted to them so they cannot be placed in any set position. We have determined 7 slots as stated in answer D.
4) Local, conditional, must be true. B is the answer.
Make local diagram with M in front row, written off to the side.
Plug in rule 4 that says that M is in front and W and Y are in back. From the main diagram we know that W can only go in 2 and S has to be in the same column.
I glanced the answers after I had the diagram and saw that S must be in the front row, because if W is in the back only S can be in the same column.
5) Local, conditional, could be true. A is the answer.
V and Y in the front row means that M could not be in the front row, as this would violate rule 4. Answer D is eliminated
So with V and Y in the front and M in the back I do a local diagram.
I did two hypotheticals with the OTP superblock (one with the block in the front row and one with it in the back row)
Since slots 1,4, and 5 are taken by OTP respectively there are only two places where V and Y can go, in slots 2 and 3, but then there is no place for either S or W to go and one of them has to be in 2 (front the main diagram) so the OTP superblock cannot go in front, it must go in back. Answers B and C are eliminated.
Only answers E and A remain so I make two hypotheticals. I already know that the OTP block must be in the back.
With OTP in the back, remember that M must also go in the back so there has to be an extra slot for it.
If R is in the back then that would mean the 1,3,4, and 5 slots are taken and M must go in 2, but either S or W must go in two so this cannot work. Eliminate E.
6) This is the drainer, but I'm ready. I know that most likely I will have to make 5 hypotheticals for this one, so I budgeted my time accordingly. D is the answer
T is the fourth column in the back row. That would mean that the OTP superblock is in the back row as well. Since R has to be in the 3rd row, either S/W must be in the second slot. R could be in the front row or the back row and either S/W can be in the slot 2 so we can't get a complete order. Eliminate A.
V is in the forth column of the front row. Since V is in the fourth slot in the front row that means that T is in the back row in the fourth slot (per rule 3) so that would mean that the OTP superblock is in the back row. R must be in the third row and S/W in the second row but we don't exactly know where these three variables go. Since we have not determined where three of the variables go we don't have a complete row settled . Eliminate B.
S is in the second column of the front row. When S is in the second column of the front row that means that W is in the second slot in the back row. But the OTP superblock can either go in the front or the back. This alone does not delineate an entire row. Eliminate C
W is in the second column of the first row. When W is in the second column of the first row then S is in the second column of the back row.
Then we look at rule 5 that says that O must be in the back row when W is in the front. Plug in the OTP superblock in the back row and slots 1,2,4, and 5 are determined. Only 3 remains. This is the trick--Since W is in front then rule 4 is violated if M is also in the front, M cannot go in the front; then W would have to go in the back and it does not. So that means that M, not in the front, must be in the back and the only place where it could possibly go is in the 3rd slot. So we have a completed row of OWMTP. D is the correct answer
Cheers,
Marty Mitchell
15 posts Page 1 of 1