Logic Challenge #7 - Feature Films

[cc4me19]

I was wondering if there could be a explanation on this one. I am out of practice and I totally blew it. Thx

[ManhattanPrepLSAT1]

[wguwguwgu]

For question4: since Q canNOT be the last film (2xOs to follow), C and E are BOTH impossible. how come E is the only choice?

Please help-thx

[noah]

For question4: since Q canNOT be the last film (2xOs to follow), C and E are BOTH impossible. how come E is the only choice?

Please help-thx

I'm not seeing why Q can't be the last. Could we have this:

Q - Q - O - O - Q

?

(sorry for the delayed response on this - I didn't get a notification about your post for some reason)

[vantagetax]

For Question 5, I only see two possible schedules:
QLPQMOMO
QMQOMLPO

What is the third possible schedule?

[timmydoeslsat]

For Question 5, I only see two possible schedules:
QLPQMOMO
QMQOMLPO

What is the third possible schedule?

QMQOMOLP

[cgschotten]

For question4: since Q canNOT be the last film (2xOs to follow), C and E are BOTH impossible. how come E is the only choice?

Please help-thx

Regarding question 4: C does work because the arrangement _ _ L _ M O _ Q complies with all rules given Q Q L P M O O Q.

Generally speaking, this game seems to be poorly constructed. I am referring particularly to rule 2 "Prestige is presented in any week immediately subsequent to a week in which Landing is presented". In other words, Prestige which must be shown at least once must be preceded by Landing (P ->LP). In that case Question#2 is an impossible scenario: "Prestige is presented in week 2 and in week 4" (_ P _ P M _ _ _). Apply rule 2: L P L P M _ _ _ and you violate rules 1 & 4.

Am I wrong on my interpretation of rule 2?

I got answers: Q1:C, Q2: impossible, Q3: no solution (because why can't this happen L P L Q M Q O O or L P Q L M Q O O...), Q4: B (violation of rule 2 by having P appear on week 1/not immediately subsequent to a presentation of Landing) & E (violation of rule 1 M L P Q M _ O Q), Q5: B (the only answer with which I agree).

[ohthatpatrick]

Yeah, I think your interpretation of rule 2 is off, and that may account for your difficulties/discrepancies.

The conditional trigger word in rule 2 is "Any", which is a sufficient trigger word.

So rather than symbolizing the rule
P -> LP
as you wrote
the rule should actually be
L -> LP

If you try the problematic questions again, I think(/hope) they will work out the way they should.

Although the wording of rule 2 is especially gnarly here, this syntax HAS come up on real LSATs.

If you check out (or remember) PT45, S3, G3, it's an In/Out game about 7 people who are in / not in a photograph together.

The first rule says
"Wendy appears in any photograph that Selma appears in"

Many people are tempted to symbolize that as
W -> S
but in reality it's
S -> W

Again, that's because "any" is the conditional word here, and "any" is a sufficient trigger word.

We could rephrase that rule as
"Any photograph that Selma appears in, Wendy appears in"

We could rephrase rule 2 from this game as
"Any week immediately subsequent to a week in which Landing is presented, Prestige is presented".

So it's not so much that the GAME was poorly constructed as it is that the evil soul of the game's writer was poorly constructed.

[wgutx08]

I also have a problem with Rule 2.

The way it is written here, I somehow understood it as "if there is a week that is immediately subsequent to L, then it is LP".

---Which would mean that, for example, L can be alone at position 8.

So I couldn't decide between A and C for Q1. For all other questions though, I had exactly same answers.

Is the language here not really tight or it was my mistake? Thanks so much for your help!

[ohthatpatrick]

I think you're reading into that too much indeterminacy.

We could rephrase rule 2 as,
Any time Landing is presented, Prestige must be presented the subsequent week.

We could say, "Cool, if Landing is in week 8, then Prestige is in week 9.", but we're constrained by only having 8 weeks, so we have to interpret this based on the finite but definite set of weeks there are. Since there is no such thing as week 9, we can't put Landing in week 8.

If Landing is in week 8, is it true that "Landing is presented in week 8"?

Yes.

If Prestige is NOT presented in the subsequent week, is it true that Prestige is presented in the week subsequent to ANY week in which Landing is presented?

No, we'd be breaking the rule.

This rule is essentially similar to
Test 51, Game 3
"Each rock classic is preceded immediately by a new composition"

This means that a rock classic can't be first to LSAT. Spot 1 isn't exempted from the rule because there 'does not exist' a spot before spot 1.

Does that make sense? I applaud your attention to detail, though.

Hope this helps.

[eligrossman6]

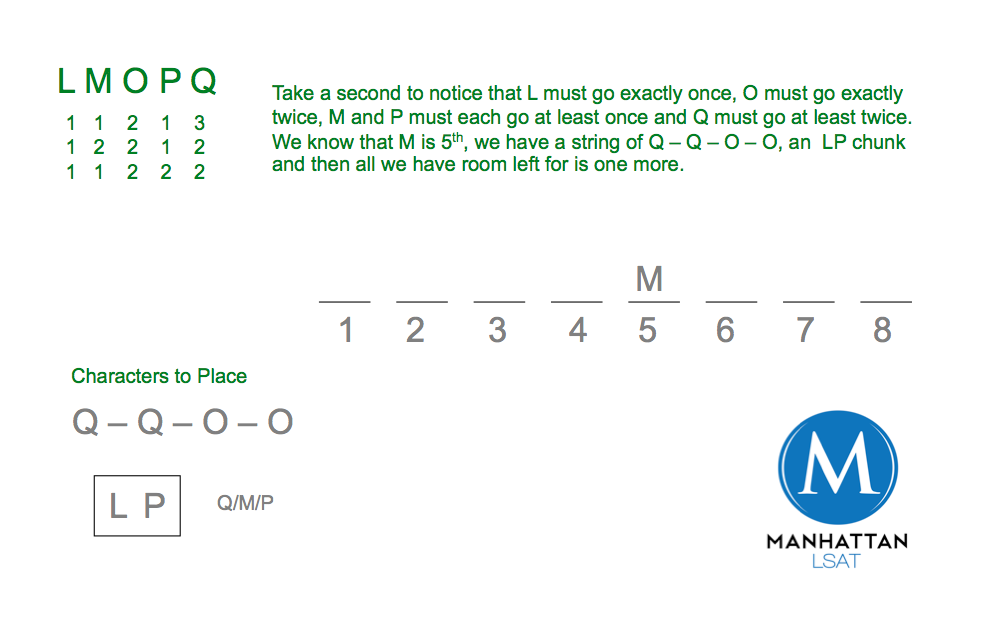
For Q5, is the only way to figure it out by trial and error (i.e. putting the second M in the open spots), or could we use the setup in some informative way?

[ohthatpatrick]

I don't know whether it's trial and error or just a lot of work, but it definitely involves quite a bit of writing.

If we know M = 2, then we know where our 8 things are coming from
M = 2
Q = 2
O = 2
L = 1
P = 1

So we have a few limitations to reconcile ..
- M is in spot 5
- we can’t have consecutive M’s, Q’s, or O’s
- both of our non-consecutive Q's come before the 2 O's
- we have an LP chunk

When I'm in doubt in an Ordering game, I lean on the chunk.

I would consider its possibilities and see if I can fill in the blanks.

L P _ _ M _ _ _
_ L P _ M _ _ _
_ _ L P M _ _ _
_ _ _ _ M L P _
_ _ _ _ M _ L P

For the first three, where the LP is before the M, we know that the two O's must come AFTER spot 5, and since they have to be split up, it's 6 and 8.

L P _ _ M O _ O
_ L P _ M O _ O
_ _ L P M O _ O
_ _ _ _ M L P _
_ _ _ _ M _ L P

Still focusing on the first three, we have to squeeze in two non-consecutive Q's before the M in spot 5. That kills off the first line and the third line. In both of those, we'd have to put the two Q's consecutively (3/4 on the first line and 1/2 on the third line).

I would cross them out on my page, but here I'll just delete them.

_ L P _ M O _ O
_ _ _ _ M L P _
_ _ _ _ M _ L P

So we know how the first line would look.

Q L P Q M O M O
_ _ _ _ M L P _
_ _ _ _ M _ L P

For the 2nd and 3rd lines, we know that the last available spot in both cases will be O, because there's no way to get both O's and both Q's (non-consecutively) BEFORE spot 5.

So we have
Q L P Q M O M O
_ _ _ _ M L P O
_ _ _ _ M O L P

So what's left?
Q, Q, O, M

The Q's have to come before the O, and the M can't be in spot 4 (no consecutive repeats).

So we get
Q L P Q M O M O
Q M Q O M L P O
Q M Q O M O L P

There are a lot of scenarios to consider (5), but remember to use whatever chunk you have as the backbone of possible scenarios.

Hope this helps.

[CrystalW913]

For Question 2: Why is Milner not an option for the third spot? It's understood that Quibble and Landing can easily go there, but I see no reason offered by the rules that would prohibit Milner. What am I missing???

[DanL677]

Any suggestions for how fast this game should be completed?

[mswang7]

We could rephrase rule 2 as,
Any time Landing is presented, Prestige must be presented the subsequent week.

I'm confused. On #4 answer choice A says L=2 & P=4 which automatically breaks the chunk rule yet it's not the correct answer? Can someone help

[Laura Damone]

First, please excuse the delayed response. This one must have slipped through the cracks amidst all the craziness of the past few weeks.

Second, this questions isn't a complete and accurate list question, it's a partial and accurate list question.

So, when A gives us L in 2 and P in 4, that doesn't actually break the LP chunk because there could be a second P in slot 3.

What A gives us is this:

__ L __ P M __ __ O

In order to satisfy the LP Chunk rule, we need a P in 3:

__ L P P M __ __ O

In order to satisfy the numeric requirements of O and Q (we need exactly 2 Os and at least 2 Qs), the remaining slots must be filled with 2 Qs and another O. The ordering rule tells us that we don't have Os until all the Qs have gone. So, our final diagram for A looks like this:

Q L P P M Q O O

Hope this helps!