Logic Challenge # 8 - City Visit

[jrdn_pearl]

can someone help me with numbers 3, 4 and 5?


thanks

jp

[dan]

Hey JP,
Attached you'll find a diagram and explanations for #3, 4, and 5.

Let me know if you have any other questions. Hope this helps.

dan

[jrdn_pearl]

dan thanks a million...and thanks for the instruction on the recorded sessions.

[ManhattanPrepLSAT1]

Here's a setup for this game!

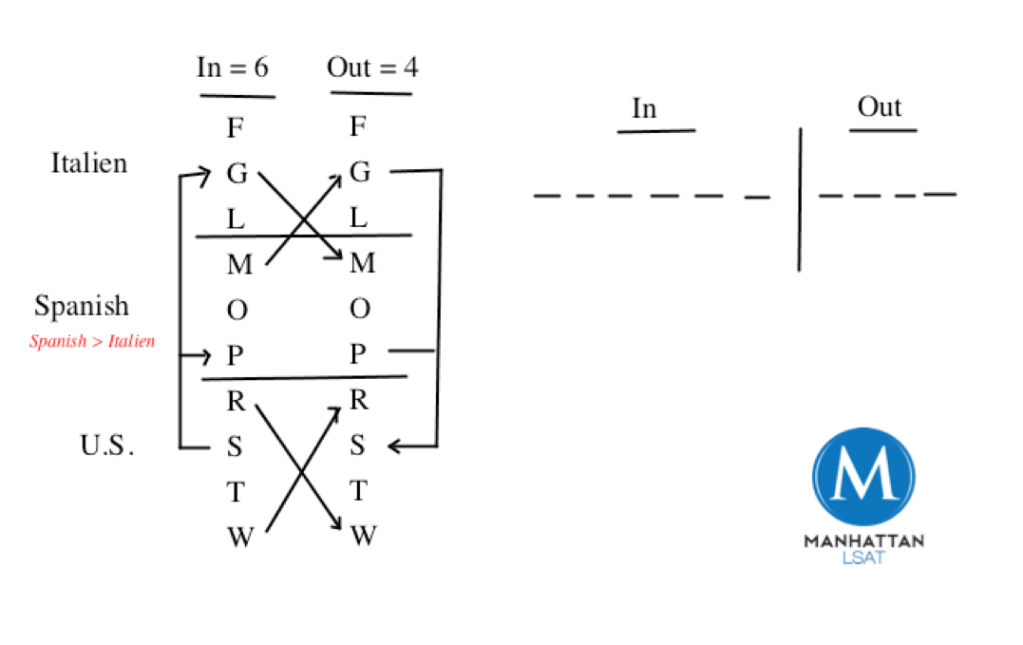
[ManhattanPrepLSAT1]

And for those of you who see that the number of cities from each country is very limited, you could even set up frames!

[ivette.sanchez1]

Hi,

Can you please re-post the explanation for #4?
Thanks!

[timmydoeslsat]

Until Matt reposts #4, I will offer my perspective on it.

It is a local question asking how many different ways we can have the six cities be sequenced with G being selected.

We know that G is an Italian city and that it forces M out, which is a Spanish city.

G _ _ _ _ _ ....M _ _ _

We know that our S's must outnumber our I's. We know that we cannot have all 3 I's in this game because how could we ever have 3 S's outnumber that?

Since we already have G (an I) we will either have G be the only I represented or it will be accompanied with exactly 1 other I.

I would show those 2 frames.

Frame with G as the only I

G _ _ _ _ _ ....M F L _

We also know that one of the out spots must be reserved for one our United States cities, either R/W

G _ _ _ _ _ ....M F L R/W

Thus everybody else must be in.

G S P T O W/R ....M F L R/W


Frame with G being with one other I

G F/L _ _ _ _ ....M _ _ _

For this scenario of 2 I's being in, we must have all 3 S's in for it to outnumber the I's. As you can tell, this is impossible with M being out. (M is out due to G being in according to this local rule given in the question stem)

This means that we only have 2 ways the cities can be ordered. One way with R being in and another way with W being in the game.