Hi,
Could you please tell me why the answer for the question below is D and not A?
LSAT Forum
2 postsPage 1 of 1
-

- bryanboone1081
-
Thanks Received: 0
-
Vinny Gambini

- Posts: 2
- Joined: August 18th, 2011
Q10
Last edited by bryanboone1081 on Thu Sep 01, 2011 11:02 am, edited 1 time in total.
-

- timmydoeslsat
-
Thanks Received: 887
-
Atticus Finch

- Posts: 1136
- Joined: June 20th, 2011

- Most Thanked

- First Responder
Re: Q10
Sure no problem. I would recommend, however, that you edit the post you made because the LSAC can become angry when individuals post copyrighted material, such as a whole game.
To answer this question effectively, you must have an effective diagram.
The diagram I came up with looks like this, and I will explain how I came up with it.
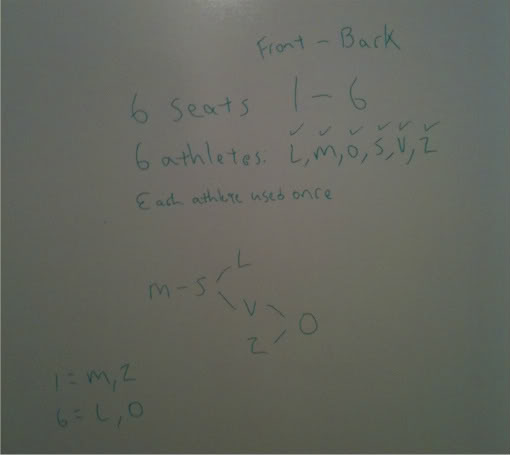
We know that we have six athletes and that each will be used exactly once. These six athletes will take up six slots, numbered 1-6. The "1" indicates the "front" and the "6" indicates the "back" of the boat.
The first rule tells us that M is closer than S. So we can show that as M - S
Notice that we do not know that M is immediately in front of S, simply that M is in front of S as of now.
Second rule is that S is closer to the front than L and V. So we can show that as well as shown by the diagram posted above. Notice that we do not know the order of L and V. We simply know that they both come after S and that is all we know.
The last rule tells us that V and Z are closer than O.
Notice that this is the first time Z and O have popped up in our rules.
Z could be first in our set up. We do not have any rules that state that a variable must come in front of Z, simply that O must come some time after it.
The tree diagram is shown above. It is important to know how to read the tree diagram. We do not know an exact order of any of the variables as of this moment. We do know that each variable is accounted for, so we do not have any floaters or randoms to keep track of.
It is important in situations like this to make a note of which variables can go first and which can go last.
I have that shown in my diagram. To see which variables can go first, simply look at the variables that do not have a variable that must come in front of it, thus that variable can go first.
And vice versa for the last spot. If no variables must go behind it, then that variable in question could go last.
This question asks us how many different spots Z could occupy.
We know that Z cannot occupy six different seats. This is because Z must come sometime before O. Thus, Z could never go last. However, Z can go 1-5. It has no rules that say that a variable must go in front of it. The only restriction of Z is that O must come at some point after it. Thus, Z can go in five different spots.
To answer this question effectively, you must have an effective diagram.
The diagram I came up with looks like this, and I will explain how I came up with it.
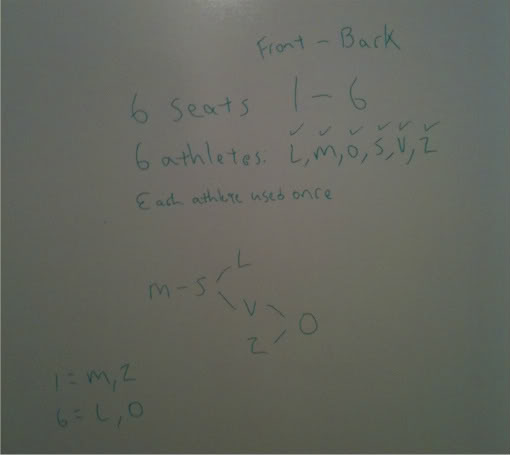
We know that we have six athletes and that each will be used exactly once. These six athletes will take up six slots, numbered 1-6. The "1" indicates the "front" and the "6" indicates the "back" of the boat.
The first rule tells us that M is closer than S. So we can show that as M - S
Notice that we do not know that M is immediately in front of S, simply that M is in front of S as of now.
Second rule is that S is closer to the front than L and V. So we can show that as well as shown by the diagram posted above. Notice that we do not know the order of L and V. We simply know that they both come after S and that is all we know.
The last rule tells us that V and Z are closer than O.
Notice that this is the first time Z and O have popped up in our rules.
Z could be first in our set up. We do not have any rules that state that a variable must come in front of Z, simply that O must come some time after it.
The tree diagram is shown above. It is important to know how to read the tree diagram. We do not know an exact order of any of the variables as of this moment. We do know that each variable is accounted for, so we do not have any floaters or randoms to keep track of.
It is important in situations like this to make a note of which variables can go first and which can go last.
I have that shown in my diagram. To see which variables can go first, simply look at the variables that do not have a variable that must come in front of it, thus that variable can go first.
And vice versa for the last spot. If no variables must go behind it, then that variable in question could go last.
This question asks us how many different spots Z could occupy.
We know that Z cannot occupy six different seats. This is because Z must come sometime before O. Thus, Z could never go last. However, Z can go 1-5. It has no rules that say that a variable must go in front of it. The only restriction of Z is that O must come at some point after it. Thus, Z can go in five different spots.
2 posts Page 1 of 1